The solar declination angle is a concept of astronomy, but its understanding is also helpful in solar energy and photovoltaic (PV) systems.
Solar declination angle calculator
Select the date, time, and your time zone to compute the declination angle in the degree.
What is the solar declination angle?
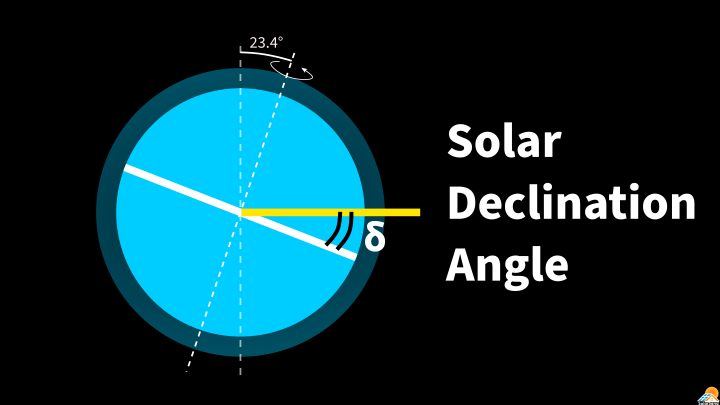
The solar declination angle is the angle between the rays of the sun and the equator of the earth. It is usually denoted by “δ.” Solar declination angle is very useful in calculating the sun elevation and the azimuth angle.
The diagram below explains the same.

Earth rotates around its own axis. It is what makes days and nights. Also, it revolves around our sun and has its own orbit.
As we might think, the earth’s axis of rotation is not perpendicular to the direction of the orbit. Instead, it has a tilt of around 23.44° (see the above figure). Because of this tilt, the equator of the earth and the rays of the sun always make some angle between them. It is what we called the solar declination angle. Other terms used for it are the sun declination angle or the declination angle of the sun.
If the earth were not tilted, the declination angle would be zero.
Declination angle with time
The solar declination angle changes time-to-time; it is not a constant number. It will be different every single day. However, the angle restricts itself between 23.44° and −23.44°. It oscillates between these two numbers; it will never cross 23.44° or fall behind −23.44°. Thus, we can conclude, the maximum value of the solar declination angle is 23.44°, and the minimum is −23.44°.
The declination angle becomes zero twice a year. It happens on March 20th and September 22nd (or 23rd). On these days, the earth’s axis of rotation becomes perpendicular to the rays of the sun. And the angle between the equator and the sun’s rays reduces to zero. These two days are called equinoxes.
In the northern hemisphere, the March equinox (March 20th) is called the spring or vernal equinox, and the September equinox (September 22nd (or 23rd)) is called the fall or autumnal equinox. In the southern hemisphere, the reverse is true.

The solar declination angle reaches a maximum on June 20th (or 21st). This day is named the June solstice. On this day, the earth is in the north of the sun. The value of the declination angle is 23.44°. In the northern hemisphere, this day is called the summer solstice, while in the southern hemisphere is called the winter solstice.
The value of the declination angle is −23.44°, the lowest, on December 21st (or 22nd). This December solstice is called the winter solstice in the northern hemisphere, whereas in the southern, it is the summer solstice.
Declination angle for your location
The declination angle is almost independent of your location. For a given UTC time, it is the same throughout the globe. It will not change from place-to-place for the same UTC time. So, whether you are in Los Angeles, Phoenix, Denver, or Boston, the solar declination will be the same for the same UTC time. However, if we include local time zones, then the declination varies slightly.
Solar declination angle formula
We can calculate the solar declination angle using the following formula:
In the above formula, d is the number of days since January 1st (UTC 00:00:00). For example, On March 3rd (UTC 00:00:00), d = 31 + 28 + 2 = 61. On December 31st (UTC 00:00:00), d = 364.
The value of δ is maximum (23.44°) when the cosine is equal to the negative one. It occurs at d = 172.5, which is around June 21st (summer solstice). The value of δ is minimum when the cosine is equal to the positive one. At d = 355 (December 21st), the cosine equals the positive one, and δ = −23.44°.
Note to consider, this formula is not very accurate and always gives a marginal error. A better formuation is mentioned in the following section.
Similarly, δ approaches zero (at d = 263.75 and 81.25) on both March and September equinoxes.
The previous formula is the simplification of the below equation.
At lower values of θ in radians, sin(θ) ≅θ. So, in the above equation, sin(θ) ≅ θ and sin(−23.44°) ≅ −23.44°.
These equations assume the earth orbits around the sun in a perfect circle.
The graph below represents the variation in the solar declination angle with time.

As we can see from the above graph, the solar declination angle is positive from the vernal equinox to the autumnal equinox—i.e., March 20th to September 22nd (or 23rd). And it is negative for the rest of the portion of the year.
Better formula
The following equation gives a more accurate value of the declination angle.
How to calculate the solar declination angle?
We can calculate the solar declination angle for a particular time from the former formula. Although the value will not be 100% accurate and a small error in the result is expected, that is acceptable for practical purposes. We only require the number of days (d) to know the angle. The table below lists some examples.
| Date (UTC 00:00:00) | Days (d) | Solar declination angle |
|---|---|---|
| January 8th | 7 | −23.24° |
| January 19th | 18 | −21.40° |
| February 16th | 46 | −13.33° |
| March 16th | 74 | −2.05° |
| March 22st | 80 | 0.53° |
| April 16th | 105 | 10.89° |
| May 16th | 135 | 20.41° |
| June 22st | 172 | 24.25° |
| July 16th | 196 | 21.31° |
| August 16th | 227 | 12.06° |
| September 23nd | 265 | −3.73° |
| October 16th | 288 | −12.94° |
| November 16th | 319 | −21.79° |
| December 22st | 355 | −23.96° |
Effect of the solar declination angle on PV systems
To make things simple, we will assume you live in the US or a country from the northern hemisphere.
Estimating the declination angle is necessary to determine the solar elevation. The solar elevation increases with the declination angle, and it is highest in months of summer. In other words, the sun moves over our heads in summer. It is the reason why in summer, we tilt our solar panels at lower angles.
As winter approaches, the declination angle goes negative, and the solar elevation decreases. Thus, in winters, the sun descends toward the horizon. Because of that, the tilt angle of solar panels increases, and the solar panels are placed almost vertical to maximize solar power.
FAQs
When is the declination angle negative?
The declination angle is negative from September 22nd (Autumnal equinox) to March 20th (spring equinox).
Why does the declination angle differ with location?
The declination angle does not differ with location for the same UTC time. However, it differs slightly with local time zones.
What is the declination angle for the winter solstice?
In the northern hemisphere, the declination angle for the winter solstice is −23.44°, while in the southern hemisphere, it is 23.44°.
What is the declination angle for the summer solstice?
In the northern hemisphere, the declination angle for the summer solstice is 23.44°, while in the southern hemisphere, it is −23.44°.
What is the solar declination angle at the vernal equinox?
The solar declination angle at the vernal equinox is 0°.
What is the angle of declination on the March equinox and September equinox?
The declination angle is zero for both March and September equinoxes.
How to find the declination angle for given coordinates?
You do not need coordinates to find the solar declination angle since it is independent of the location. You only need the date and time.
Does the solar declination angle change daily?
Yes, the solar declination angle does change daily. But the change is practically insignificant in a short period of time.
What happens to the angle of declination as you get closer to the poles or the equator?
Whether you are at the poles or the equator, the angle of declination will not change for the same UTC time. It is the same across the earth for a given moment of time.